Jump to:
Using Hands-On Material
You can use hands-on materials to introduce systems of equations, helping both braille reading and sighted students to better understand what a system of equations is and how to solve it.
We will demonstrate two examples using screws, nuts and washers to represent the variables x, y and z. Have students create different combinations of screws, nuts, and washers, and then weigh each combination. If you don’t have a balance scale, simply provide the students with the weights for each combination. The goal is for students to identify the corresponding system of equations and then solve it.
Example 1
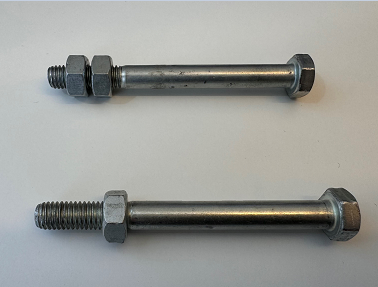
The weight of one screw and two nuts is 120 grams. The weight of one screw and one nut is 110 grams. Written with words and with mathematical symbols, the system of equations is:
Screw + 2 nuts = 120 grams
Screw + nut = 110 grams
x + 2y = 120
x + y = 110
Example 2

The weight of one screw and three nuts is 130 grams. The weight of one screw and one nut is 110 grams. The weight of one screw, two nuts and two washers is 130 grams. Written with words and with mathematical symbols, the system of equations is:
Screw + 3 nuts = 130 grams
Screw + nut = 110 grams
Screw + 2 nuts + 3 washers = 130 grams
x + 3y = 130
x + y = 110
x + 2y + 3z = 130
Graphical Solution
For braille readers, solving a system of equations graphically can be time-consuming. Despite this challenge, graphical solutions provide valuable mathematical insights into the relationship between graphs and equations, as well as the graphical interpretation of solving a system of equations. Braille readers can utilize tactile graphs on paper or programs like Desmos. For more details, refer to the instructional guide on graphs for math teachers.
Algebraic Solution
Most braille readers prefer to use the substitution method over the elimination method when solving systems of equations algebraically. This is due to the lack of overview and the increased difficulty of manipulating the equations by navigating between them.
Sighted students often label the equations in the system as 1, 2 etc. To prevent the braille reader from inadvertently perceiving these numbers as part of the equation, they can choose not to use numbers. Use Roman numeral symbols such as i, ii etc.
Example
Solve the following system of equations: 2x ‐ y = 8 3x + 4y = 1
In the following solution, the notation “(a)”, “(b)” etc. refers to the explanation below. The student should not use this notation.
(a) i: 2x ‐ y = 8
(b) ii: 3x + 4y = 1
(c) i: y = 2x ‐ 8
(d) ii: 3x + 4(2x ‐ 8) = 1
3x + 8x ‐ 32 = 1
11x = 33
x = 3 ‐ ‐ ‐
(e) y = 2 * 3 ‐ 8 = 6 ‐ 8 = ‐2 ‐ ‐ ‐
(f) Solution: x = 3 and y = ‐2===
Explanation
(a) and (b) Number the equations and line them up on separate lines (from margin). Copy and paste both equations on the two lines below.
(c) Isolate y in the first equation
(d) In the second equation (ii) substitute for y its equivalent expression and solve this equation.
(e) Insert the value for x (=3) in equation i. Copy the expression and paste it on the same line, and edit/replace x with 3 in the copy.
(f) Write the final answer
Alternatively, the student writes the different terms one after another, separating them with spaces or semicolons.
i: 2x ‐ y = 8; ii: 3x + 4y = 1
If the expression is longer than what fits on the braille display, it can be more difficult to get an overview and to manipulate the expressions.
